20-May-2015: Conservation of Energy/Conservation of Angular Momentum

Purpose:

To release a meter stick, pivoted at one end, so that it swings and collides inelastically with a blob of clay and to record the height the clay at the end of the stick reaches.

To find the theoretical height the clay reaches using calculations from conservation of energy and conservation of angular momentum.

To compare these results.

Set-Up:

The apparatus consist of a meter stick hanging from one pivoted end and piece of clay with tape of negligible mass wrapped around it so that the meter stick inelastically collides with the clay.

Clay lined up so that it's cm is nearly at the very tip of the stick

Experiment:

1. First we needed to collect all the measurable data such as the weight of the clay and meter stick at its center of mass.

Mass of Clay = 25.3 grams

2. To perform the experiment we lifted the meter stick until it was parallel to the ground and set our pivot point as our GPE=0.

3. We then recorded, on video capture, the dropping of the meter stick going from full GPE to all KE and then inelastically colliding the piece of clay, rising a specific height. 

Data Analysis:

1. To start, we analyzed the video capture data to figure out how high the meter stick raised after inelastically colliding with the piece of clay, shown below: 

Height of clay reached above the ground = 0.2904 meters

2. Next we needed to find the theoretical height the clay would reach through analyzing the scenario through three separate phases.

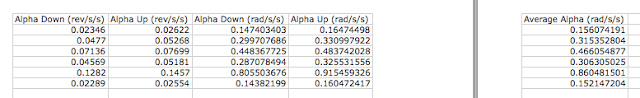
First, we looked at the switch of energy from potential to kinematic of the meter stick to find the angular velocity of its center of mass, just before the collision with the clay. 

Omega of Ruler = 6.067 rad/s

Second, we used this omega as the initial omega and used momentum to find the final omega after the collision of the mass and clay. 

Omega of Clay and Ruler = 3.170 rad/s

Lastly, we looked at energy again to find the angle theta that the meter stick would rotate, using that to find the theoretical height reached by the clay after the collision.

Theoretical height reached by clay = 0.401 m

% Error: 

|(Theoretical height-Actual height reached by clay)|/Theoretical height *100% = percent error

|(0.401-0.290)|/(0.401) * 100% = 27.58%

Conclusion:

When finding the theoretical height through conservation of energy and angular momentum we estimated the clay to rise 0.401 meters off the ground when in reality it rose 0.290 meters. Although our percent error was roughly 25% off, it was not surprising the actual height reached would be less than predicted. Although the energy was conserved, there were many factors not taken into account when finding the theoretical height, such as loss of energy due to heat and friction of the pivot (let alone human error such as not using the correct number of sigfigs). The experiment was successful is proving to us the conservation of angular moment and conservation of energy. 

18-May-2015: Moment of Inertia and Frictional Torque of the Big Wheel

Purpose:

Part 1: To find the frictional torque of the big steel wheel.

Part 2: To use this torque to find inertia of the wheel, that'll be used to then predict the time it takes for a cart to drop a meter that is attached to the wheel by a string wrapped around it.

Part 1: Finding the Frictional Torque

Set-Up:

The wheel being turned actually has three parts, the outside cylinders and the main wheel in the middle.

Experiment:


Over all, to find the frictional torque we need to find the angular deceleration of the wheel and the Inertia of the wheel.

1. To find the angular deceleration we set up a video capture on logger pro, using the camera shown above. This video would then be analyzed to find the angular deceleration of the wheel after we spin it.

2. To find the moment of inertia, we needed to measure the radius and mass needed, and then calculate it.

Data Analysis:

1. Using the video we marked every revolution recording it's time with video analysis, giving us revolutions per seconds or omega.

Blue Dots Represent Each Revolution

Next then plotted omega vs time to find the slope as our angular deceleration (where omega was translated into rad/s):

Angular Accerlation = -0.3768 rad/s/s

2. Since inertia depends on mass and how far it is from the center of rotation, we had to find the radius of each cylinder and the according mass shown below:

Since inertia for a disk is  I=.5mr^2, we were able to plug our values of radiuses and masses to find the inertia of the rotation apparatus:

Now that we had inertia and angular acceleration, we are able to find the frictional torque using Torque = Inertia * Angular Acceleration.

(where the y= represents the translation from slope found above into alpha)

Part 2: Finding time of Cart Going Down a Slope for 1 Meter

Set-Up:

We set up a ramp in which the cart attached to a string would roll down. The string would also be wrapped around the big wheel, rotating the whole mass of the big wheel and the two cylinders on each side:

Experiment:

We then recorded the measurable data needed in calculating a predicted time, recording the angle the ramp was at and the weight of the cart.

We then ran the cart down the ramp for one meter three times each and recorded the time it took.

Data Analysis:

We then calculated the predicted, "true" value of the time the cart should take in rolling down the meter ramp by taking the torque of the cart and subtracting the frictional torque and setting that equal to the inertia of the big wheel and it's angular acceleration. Since the acceleration was the unknown that would help us find time, we had to derive an equation to find it using the force equation of the cart and assuming that the acceleration of the cart and the tangential acceleration of the big wheel were the same. Calculations show below:

If you look above, the experimental values are also shown below the calculated time of 7.34seconds.

Conclusion:

In considering factors such as assumptions being made like acceleration of the cart being exactly the same as the tangential acceleration of the wheel as well as a possibly incorrectly recorded frictional torque (uncertain due to possible measuring errors and inaccurate video analysis), we compared our results:

With a percent error less than 5% we successfully completed our experiment, realizing how to find frictional torque and a mass' inertia and using these properties to find the tangential acceleration of that mass if a torque was applied it. 

14-May-2015: Moment of Inertia of a Uniform Triangle

Purpose:

To experimentally determine the moment of inertia of a right triangular this plate around its center of mass, for two perpendicular orientations of the triangle.
To then determine the "true" moment of inertia through calculations.
To compare our results.

Set-Up:

We mounted on a holder and disk onto the rotating system. The upper disk floated on a cushion of air to avoid friction. A string was wrapped around a disk attached to the upper disk and the triangle so that when the other end of it fell with a light mass, the string unraveled and turned the system providing torque. The torque pulley, disk and holder are shown below:

System without Triangle

We would then add the triangle onto the holder:

System with Triangle

Experiment:

1. First we set up the experiment so that we could find the angular acceleration of the rotating disk and holder when the light mass is dropped and provides a torque. Therefore, this will be used to find the inertia of the system without the triangle.

Angular Acceleration of Upper Steel Disk and Holder

2. Next we will need to collect the measurable data which included the mass of light mass, radius of the turning disk where the torque occurs, length, width, and weight of the triangle:

Mass of Light Hanging Mass

Radius of Disk with Torque

Width of Triangle

Length of Triangle

Mass of Triangle

Overall Recorded Measured Data: 

3. Next we performed the first step of the experiment so that we could find the angular acceleration of the rotating disk and holder with the triangle vertical when the light mass is dropped and provided a torque.

Angular Acceleration of Upper Steel Disk, Holder, and Vertical Triangle

4. Lastly, we recorded the angular acceleration with the triangle horizontal. 

Data Analysis: 

1. First we need to organize our angular accelerations. To find the angular acceleration we looked at the slope of the graph of angular velocity. Realizing that there is still slight friction between the steel disc even with an air cushion, we needed to take the average:

(rad/s/s)

2. Now that we have angular acceleration we need to know it's relationship to Inertia:

Torque = Inertia * Angular Acceleration

We are able to find the experimental Inertia of the Triangle by subtracting:

Inertia of System with Triangle - Inertia of System without Triangle = Inertia of Triangle

Using the same method from the previous experiment, we used our derived equation to find Inertia:

Next we are able to find our experiment's Inertia equation using the measured data:

mass of light mass = 24.5g (switch g to kg in the picture)
radius of disk with torque = 2.25cm

Experimental Inertia's found:

3. Lastly, we found "True" Inertia's of the vertical and horizontal triangle.

We first had to find the moment of inertia for a triangle being spun around it's edge:

Next we used Euler's law of motion to find the moment of inertia of a triangle rotated about its center of mass:

Using this equation we were able to plug in the vertical and horizontal data to find the "true" moment of Inertia:

Conclusion:

Comparing the results of the experimental inertias to the "true" calculated interias, we found this percent error:

This shows that our experimental values and calculated values were very close, a successful data analysis. Although uncertainties may have occurred with taking the measurable data or not getting a close enough angular acceleration reading, our results proved that moment of inertia can be found through correct integration, just like we have been doing in class, and reflect "real-world" experimental results.   

4-May-2015: Angular Acceleration

Purpose:

Part 1:
To find the angular acceleration of spinning objects (in this case disks).
To use this data to find the relationship between linear acceleration versus angular acceleration, observe the effect of changing the hanging mass, effect of the radius on which the hanging mass exerts a torque, and the effect of changing the rotating mass.

Part 2:
To compare the theoretical moment of Inertia to the experimental moment of Inertia.

Set up:

We wanted to apply a known torque to the rotating disk so we used a string wrapped around the small disk, above the larger one, to be pulled by a hanging mass off of a pulley, creating a known torque and a measurable rotational acceleration of the disk. To measure the rotational acceleration, we used equipment that recorded this acceleration by counting the lines marked on the sides and converting the data onto a graph of angular acceleration on the table. Air flow between the two large disks allowed only the upper disk to be rotated with the small disk that had the string wrapped around it.

Air flow between the two steel discs makes them almost frictionless

Hanging mass being dropped

Free Body Diagram:

Therefore, an average of the descending and ascending angular accelerations must be found for alpha. 

We performed this experiment six different time in six different ways:

Experiment 1:

We let the mass drop and recorded the angular acceleration as the 25 gram mass dropped down and back up again, shown below: 

Hanging Mass Only, Small Torque, Top Steel Disk Angular Acceleration

We also recorded the linear acceleration for the first experiment:

Hanging Mass Only, Small Torque, Top Steel Disk Linear Acceleration

Experiment 2: 

Angular acceleration with the same set up as experiment 1 but with a hanging mass with twice the hanging mass:

2x Hanging Mass, Small Torque, Top Steel Disk Angular Acceleration

Experiment 3:

Angular acceleration with the same set up as experiment 1 but with a hanging mass with three times the hanging mass: 

3x Hanging Mass, Small Torque, Top Steel Disk Angular Acceleration

Experiment 4:

Angular acceleration with the same set up as experiment 1 but with a larger torque due to a larger "massless" disk creating a larger radius on which the hanging mass' string is wrapped around:

Hanging Mass Only, Large Torque, Top Steel Disk Angular Acceleration

Experiment 5:

Angular acceleration with the same set up as experiment 4 but with an aluminum top disk being rotated affecting the rotating mass:

Hanging Mass Only, Large Torque, Top Aluminum Disk Angular Acceleration

Experiment 6: 

Angular acceleration with the same set up as experiment 4 but with both the top and bottom steel discs being rotated affecting the rotating mass:

Hanging Mass Only, Large Torque, Top Steel + Bottom Steel Disk Angular Acceleration

Data Analysis of Part 1: 

Angular Accelerations Collected from Experiments 1-6:

Alpha Data Table in rad/s/s

Overall Data:

Relationship between angular acceleration and linear acceleration:

alpha = angular acceleration 

a= linear accelertion

(hmmm my calculations dont look to good....PIC)

Effects of changing the hanging mass with Experiments 1, 2, and 3:

Hanging mass of Experiment 1 = 24.6 grams

Hanging mass of Experiment 2 = 49.6 grams

Hanging mass of Experiment 3 = 74.6 grams

where m = hanging mass = about 25grams 

Effects of changing the radius on which the hanging mass exerts a torque with Experiments 1 and 4:

Diameter of the small "weightless" disk: 2.5cm 

Diameter of the large "weightless" disk: 5.0cm 

where r = d/2 

Effects of changing the rotating mass with Experiments 4, 5, and 6:

Rotating Mass of Experiment 4:

Mass of Top Steel Disk = 1.3481 kg

Rotating Mass of Experiment 5:

Mass of Top Aluminum Disk = 0.455 kg

Rotating Mass of Experiment 6: 

2x  = 2.696 kg

Mass of Top and Bottom Steel Disk

Relationship between rotation mass and the angular acceleration:

Data Analysis of Part 2: 

Measured Data:

• the diameter and mass of the top steel disk: 12.65cm +- .002, 1.348kg +- .001kg

Diameter of Steel/Aluminum disks: 12.65 cm +- .002

• the diameter and mass of the bottom and steel disk: 12 cm, 2.696 kg +- .001kg
• the diameter and mass of the top aluminum disk: 12cm, 0.455 kg +- .001kg
• the diameter and mass of the smaller torque pulley: 2.5 cm +- .02cm, 9.9g +- 0.1g

Mass of Small Disk = 9.9 g

• the diameter and mass of the larger torque pulley: 5.00cm +- 0.02cm, 36.0g +- 0.1g

Mass of Large Disk = 36.0 g

• the mass of the hanging mass supplied with the apparatus: 24.6g +- 0.1g

Mass of Hanging Mass = 24.5 g +- 0.1g

Overall, our set of measured data is shown below:

Although the relationship between the angular accelerations in part 1 remain the same, we had to multiply them by a factor, since we recorded the data in the wrong setting on Logger Pro.  This is for a more accurate set of data of the angular accelerations shown below:

Now that the data from part one is organized and accurate, we have all the data necessary in calculating the experimental inertia and comparing it to the theoretical inertia.

Equations

Experimental Moment of Inertia:

Derived in class and on the handout, we used the free body diagram of the hanging mass and the experimental angular accelerations to find the reduced equation.

Free Body Diagram

If there was no friction in the system (taken account for by finding the average angular acceleration), then the experimental equation would by how it is shown below:

Theoretical Moment of Inertia:

The theoretical calculation of a disk's inertia is:

I = (1/2)MR^2

Actual Calculations Compared:

(since data was changed, calculation of inertia edits credited to Alysia Lukito)

% Error:

In comparing inertias, we were looking at the inertia of the steel disk, aluminum disk, and the two disks combined. For the first % error of the top steel disk, the average was taken from experiments 1,2,3, and 4 while the second % error is from experiment 5 for the aluminum upper disk, and the third % error was from experiment 6 for both disks combined. 

(Percent Error was edited as well by Alysia Lukito)

Conclusion:

In the data analysis of part 1 each of the three sections had different reasons for not having an exact relationship. When looking at the different hanging masses of experiments 1-3, the masses were not exact multiples of each other, which meant that the angular accelerations of each experiment would be close but not be exact multiples of each other as it is in theory for determining their relationship. When looking at the radius, the relationship fit with little percent error although sigfigs could still have been a factor. When looking at the different torque, incorrect mass may have been a factor in assuming the top steel disk and the bottom steel disk were equal mass.

In the data analysis of part 2 we see very small, under 5 %, error in the experimental inertia versus the theoretical inertia of the top disks. When comparing the inertia of the disks combine we seem to have a 28% error in which we agreed may have been caused by friction unaccounted for by the axis or not putting the cap on tight enough to avoid friction of the two plates with the surface below.

In the end we were proven that T=Fr=I(alpha), confirmed in the first part by deriving different manipulations of the equation that proved this relationship accurate of the different angular accelerations recorded after changing their radius, mass, and torque. We then proved this relationship in finding inertia and using the derived formula of inertia for a disk 1/2 MR^2 found by integral, which were almost the same for the aluminum disk and steel disk.