2-April-2015: Centripetal force with a motor

The apparatus shown below has a motor that spins at an angular speed w. The mass attached at the end of the string is therefore revolved around a centripetal shaft with a radius that increases as the angle alpha increases. The first goal is to find the relationship between w and alpha.

Apparatus at rest

Apparatus in motion

To find w:

1. We first found alpha from looking at the right triangle with hypotenuse L and height 2 which we found by measuring the distance the spinning object was from the ground and subtracting that from the height of the entire apparatus.

2. The height of the spinning object from the ground was estimated by using the tool shown below, where we recorded the height as soon as the spinning object just barely grazed the top of the attached and projected piece of paper. 

Used to find height of spinning object above the ground

The data from trial one found using these methods are written in red below. The blue is the derived formula for w that uses the variables we just discussed. This is an example of how we used height to find w. 

Calculations to find omega (w)

We then collected multiple values of h at a variety of values of w by increasing the voltage of the motor driving the system's angular speed. To test this model, we then made it our next goal to test the accuracy of these results. We decided to line up our values of omega found by using height with values of omega found by using time. 

Our Data Results:

Each recorded height and time used to find omega

The data shows the time t that it took for one revolution (found by taking the time of ten revolutions and dividing that number by ten). The calculations for finding omega according to the period are clearly shown in each trial. Next we found omega according to the recorded height. 

Calculations used to find omega with height

After using logger pro to also calculate our data, we created a data table with each omega found from each trial. We then plotted the results of both omegas of each trial against eachother in hopes of similar answers or in graphical terms, a slope of 1. 

This was our resulting graph: 

Our results proved successful with a slope of 0.9713. Although these results were close, we had to account for range of error possible in taking the data. After looking at the highest and lowest possible error in recording the time and height, we were able to calculate the highest omegas and lowest omegas from each set of data. 

For example, trial one's range of error:

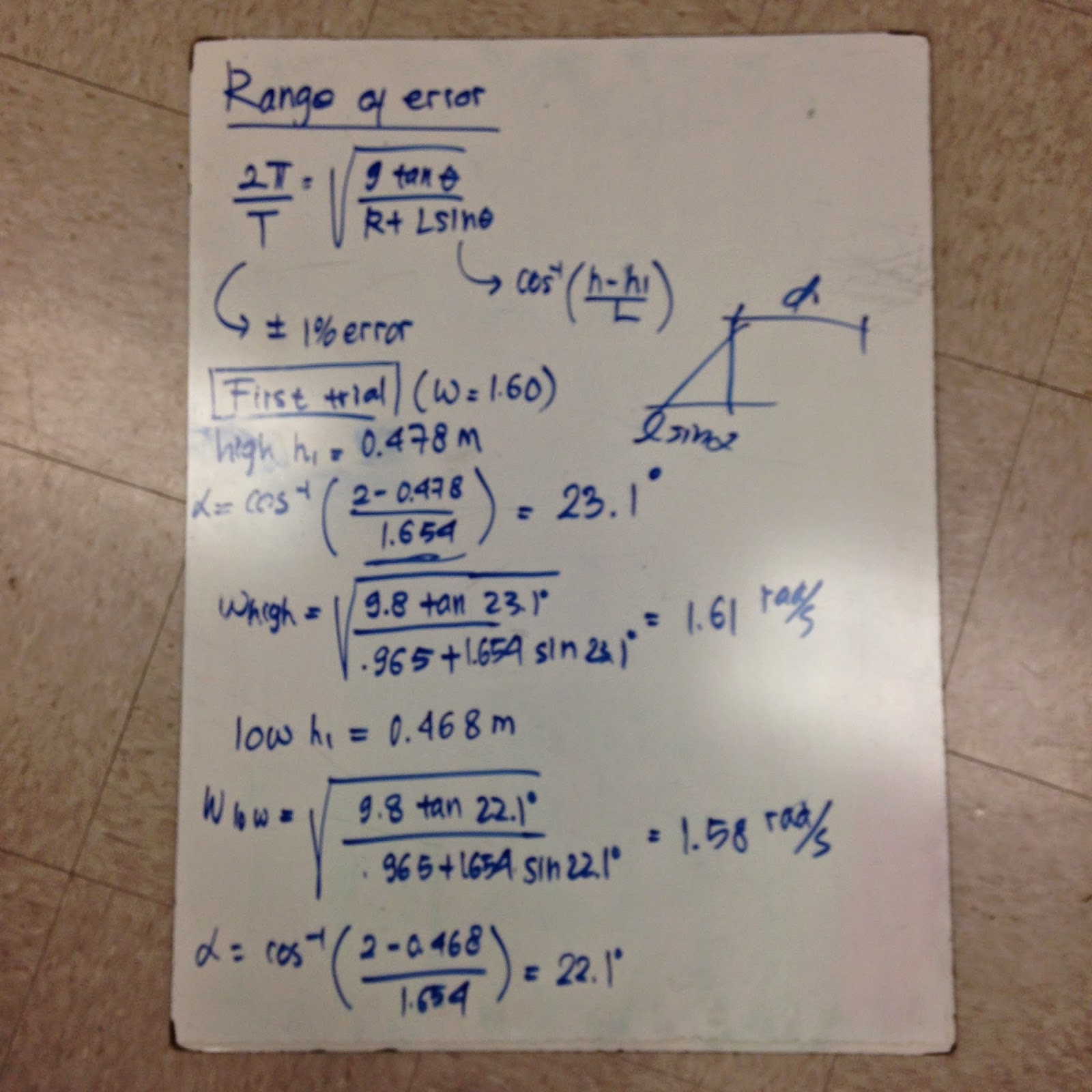Range of Error Cacluations

Using these results from the highest to the lowest possible omegas of trial one and trial six we were able to create a range of error by plotting two more graphs. 

Lowest Resutls

Highest Results

In the end, our slope ranged from 0.9824-0.9696, a reflection of a very successful experiment in calculating omega by using the relationship between angular speed and the angle caused by the centripetal force of the rotating apparatus.